A pipe can fill a cistern in 9 hours. Due to a leak in its bottom, the cistern fills up in 10 hours. If the cistern is full, in how much time will it be emptied by the leak?
एक पाइप एक टैंक को 9 घंटे में भर सकता है. इसके तल में एक रिसाव के कारण, टैंक 10 घंटे में भर जाता है. यदि टैंक भरा हुआ है, तो रिसाव से यह कितने समय में खाली हो जाएगा?
A pipe can fill the cistern in 9 hours.
The part filled by the pipe in 1 hours = 1/9
Due to leak the cistern fills up in 10 hours.
The part filled by pipe due to leak in the cistern = 1/10
The part emptied by the leak in 1 hours = The part filled by the pipe in 1 hours - The part filled by pipe due to leak in the cistern
= 1/9-1/10
= 10-9/90
= 1/90
So in 90 hours, the cistern will be emptied by the leak.
Hence the correct answer is option C.
एक पाइप टंकी को 9 घंटे में भर सकता है।
पाइप द्वारा 1 घंटे में भरा गया भाग = 1/9
रिसाव के कारण टंकी 10 घंटे में भर जाती है।
टंकी में रिसाव के कारण पाइप द्वारा भरा गया भाग = 1/10
1 घंटे में रिसाव द्वारा खाली किया गया भाग = 1 घंटे में पाइप द्वारा भरा गया भाग - टंकी में रिसाव के कारण पाइप द्वारा भरा गया भाग
= 1/9-1/10
= 10-9/90
= 1/90
अत: 90 घंटे में टंकी रिसाव से खाली हो जाएगी।
अतः सही उत्तर विकल्प C है।
A gun is fired from a fort. A man hears the sound 10 seconds later. If the sound travels at the rate of 330 m/sec, find the distance between the fort and the man.
एक किले से बन्दुक से गोली चलाई जाती है। एक व्यक्ति को उसकी आवाज़ 10 सेकंड बाद सुनाई देती है । यदि ध्वनि 330 मी./ से से यात्रा करती है , तो किले और उस व्यक्ति के बीच की दूरी बताइए।
Given -
Speed = 330 m/sec
Time = 10 sec
Distance between fort and man = Speed*time
= 330*10
= 3300 km
= 3300/1000 m
= 3.3 m
Hence the distance between the fort and the man is 3.3 m.
So the correct answer is option C.
दिया गया है -
गति = 330 मीटर/सेकंड
समय = 10 सेकंड
किले और व्यक्ति के बीच की दूरी = गति*समय
= 330*10
= 3300 किमी
= 3300/1000 वर्ग मीटर
= 3.3 वर्ग मीटर
अत: किले और व्यक्ति के बीच की दूरी 3.3 मीटर है।
इसलिए सही उत्तर विकल्प C है।
What will be the sum of (101+102+103….+200)?
(101+102+103….+200) का योग क्या होगा ?
Solution:
Total number of terms = [(last term-first term)/difference] +1
Total number of terms = [(200-101)/1]+1
= 99+1
= 100
Sum of total terms of the series = [(first term + last term /2)] x n
= [101+200/2] x 100
= [301/2] x 100
= 301 x 50
= 15050
Sum of series (101+102+103….+200) = 15050
So the correct answer is option C.
हल:
कुल पदों की संख्या = [(अंतिम पद-प्रथम पद)/सार्वांतर] +1
कुल पदों की संख्या = [(200-101)/1]+1
= 99+1
= 100
श्रेणी की कुल पदों का योग = [(प्रथम पद + अंतिम पद /2)] x n
= [101+200/2] x 100
= [301/2] x 100
= 301 x 50
= 15050
श्रेणी (101+102+103….+200) का योग = 15050
अतः सही उत्तर विकल्प C है l
The difference between a number and its two-fifth is 510. What is 10% of that number?
एक संख्या और उसके 2/5 के बीच का अंतर 510 है। उस संख्या का 10% क्या है?
Let the number = x
two-fifth of the number = x*(2/5)
According to the question - x-(2/5)x = 510
= 3x/5 =510
= x =[510 * ( 5/3)]
= x = 850
So the number is 850.
Now 10% of the number -
= 10 % 0f 850
= 85
Hence the correct answer is option B.
माना संख्या = x
संख्या का 2/5 = x*(2/5)
प्रश्न के अनुसार - x-(2/5)x = 510
= 3x/5 =510
= x =[510 * ( 5/3)]
= x = 850
अतः संख्या 850 है l
संख्या का 10% -
= 850 का 10 %
= 85
अतः सही उत्तर विकल्प B है।
A large tanker can be filled by two pipes A and B in 60 and 40 minutes respectively. How many minutes will it take to fill the tanker from empty state if B is used for half the time and A and B fill it together for the other half ?
एक बड़े टैंकर को दो पाइप A और B क्रमशः 40 मिनट और 20 मिनट में भर सकते हैं। यदि पाइप B का आधे समय उपयोग किया जाता है एवं दूसरे आधे समय के लिए पाइप A और B इसे एक साथ भरते हैं, तो खाली टैंकर को भरने में कितने मिनट लगेंगे?
Pipe A can fill the tank in 60 min.
The part of the tank filled by pipe A in 1 min = 1/60
Pipe B can fill the tank in 40 min.
The part of the tank filled by pipe B in 1 min = 1/40
The part of the tank filled by both the pipes in 1 min = 1/60+1/40=2+3/120
= 5/120
= 1/24
Let the total time taken to fill the tank is x min.
Half time = x/2 min
According to the question if B is used for half the time and A and B fill it together for the other half.
The part of the tank filled by A+B in x/2 time= x/2 * 1/24 = x/48…(1)
The part of the tank filled by pipe B in x/2 time = x*2 * 1/40 = x/80…(2)
Adding (1) and (2)
x/48+x/80 = 1 work
x/48+x/80 = 1
5x+3x/240 = 1
8x/240 = 1
x/30 = 1
x = 30 min
Hence it will take 30 min to fill the empty tank.
Hence the correct answer is option D.
पाइप A टंकी को 60 मिनट में भर सकता है।
पाइप A द्वारा 1 मिनट में भरा गया टैंक का हिस्सा = 1/60
पाइप B टंकी को 40 मिनट में भर सकता है।
पाइप B द्वारा 1 मिनट में भरा गया टैंक का हिस्सा = 1/40
दोनों पाइपों द्वारा 1 मिनट में भरा गया टैंक का हिस्सा = 1/60+1/40=2+3/120
= 5/120
= 1/24
माना टंकी को भरने में लगा कुल समय x मिनट है।
आधा समय = x/2 मिनट
प्रश्न के अनुसार यदि आधे समय के लिए B का उपयोग किया जाता है और दूसरे आधे समय के लिए A और B इसे एक साथ भरते हैं।
A+B द्वारा x/2 समय में भरा गया टैंक का हिस्सा = x/2 * 1/24 = x/48…(1)
पाइप B द्वारा x/2 समय में भरा गया टैंक का हिस्सा = x*2 * 1/40 = x/80…(2)
(1) और (2) को जोड़ने पर
x/48+x/80 = 1 कार्य
x/48+x/80 = 1
5x+3x/240 = 1
8x/240 = 1
x/30 = 1
x = 30 मिनट
अतः खाली टंकी को भरने में 30 मिनट का समय लगेगा।
अतः सही उत्तर विकल्प D है।
In the figure the shaded region represents:
चित्र में छायांकित क्षेत्र निरूपित करता है:
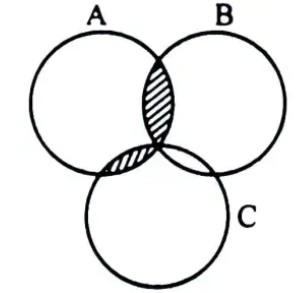
In the figure, the shaded region represents A∩(BUC).
So the correct answer is option D.
चित्र में छायांकित क्षेत्र A∩(BUC) को दर्शाता है।
इसलिए सही उत्तर विकल्प D है।
Three taps A, B and C can fill a tank in 20, 30and 40 minutes respectively. All the taps are opened simultaneously and after 5 minutes tap A was closed and then after 6 minutes tab B was closed. At the moment a leak developed which can empty the full tank in 70 minutes. What is the total time taken for the completely full ?
तीन नल A, B और C एक टैंक को क्रमशः 20, 30 और 40 मिनट में भर सकते हैं। सभी नल एक साथ खोले जाते हैं और 5 मिनट के बाद नल A को बंद कर दिया जाता है और फिर 6 मिनट के बाद नल B को बंद कर दिया जाता है। उसी समय एक रिसाव विकसित हुआ है जो 70 मिनट में पूरे टैंक को खाली कर सकता है। पूरी तरह से भरने में लगने वाला कुल समय कितना है?
Three taps A, B and C can fill a tank in 20, 30 and 40 minutes respectively.
All the taps are opened simultaneously and after 5 minutes tap A was closed.
Upto 5 min the part filled by taps A, B and C = 5(1/20+1/30+1/40) = 5(6+4+3/120)
= 5(13/120)
= 65/120
After 6 minutes tab B was closed.
Upto next 6 min the part filled by tap B and C = 6(1/30+1/40)
= 6(4+3/120)
= 6(7/120)
= 42/120
The part filled by taps A, B and C upto first 11 min = 65/120+42/120
= 107/120
Ramianing part = 1-107/120
= 13/120
Now only tap C is opened.
At the moment a leak developed which can empty the full tank in 70 minutes.
So the part filled by tap C and leak in 1 hours = 1/40-1/70 = 7-4/280
= 3/280
Tap C and leak can fill the tank in 280/3 hours.
So 13/120 part of the tank will be fill by tap C and leak = 13/120*(280/3)
= 91/6 m
So the time taken to fill the tank completely = 11+91/6 = 66+91/6
= 26.16 min
Hence the correct answer is option B.
तीन नल A, B और C एक टंकी को क्रमशः 20, 30 और 40 मिनट में भर सकते हैं।
सभी नल एक साथ खोले जाते हैं और 5 मिनट बाद नल A को बंद कर दिया जाता है।
5 मिनट तक नल A, B और C द्वारा भरा गया भाग = 5(1/20+1/30+1/40) = 5(6+4+3/120)
= 5(13/120)
= 65/120
6 मिनट के बाद नल B को बंद कर दिया गया।
अगले 6 मिनट तक नल B और C द्वारा भरा गया भाग = 6(1/30+1/40)
= 6(4+3/120)
= 6(7/120)
= 42/120
नल A, B और C द्वारा पहले 11 मिनट तक भरा गया भाग = 65/120+42/120
= 107/120
शेष भाग = 1-107/120
= 13/120
अब केवल नल C खोला गया है।
उसी समय एक रिसाव विकसित हुआ है जो 70 मिनट में पूरे टैंक को खाली कर सकता है।
तो नल C और रिसाव द्वारा 1 घंटे में भरा गया हिस्सा = 1/40-1/70 = 7-4/280
= 3/280
नल C और रिसाव टैंक को 280/3 घंटे में भर सकता है।
तो टंकी का शेष 13/120 भाग नल C और रिसाव द्वारा भरा जाएगा = 13/120*(280/3)
= 91/6 मिनट में
तो टंकी को पूरा भरने में लगा समय = 11+91/6 = 66+91/6
= 26.16 मि
अतः सही उत्तर विकल्प B है।
What was the day of the week on 28th May, 2006?
28 मई, 2006 को सप्ताह का कौन सा दिन था?
To solve this question, we can divide 28 May 2006 into small parts.
To 28 May 2006, we can write (2000 + 5 + 1 January 2006 + 28 May 2006).
2000 = 1600 + 400 = 0 odd days + 0 odd days = 0 odd days
Now we will find the number of odd days From 2000 to 2005.
Odd days in 5 years = 4 ordinary years + 1 leap year (2004 is leap year between 2000 and 2005)
Odd days in an ordinary year = 1
Odd days in leap year = 2
Odd days in 5 years = 4*1 + 1*2 = 4+2 = 6 odd days
Total number of days from 1st Jan 2006 to 28th May 2006
January+February+March+April+28 days (May days)
31 +28+31+30+28 = 148 days
Odd days in 148 days = 148/7 = 21 + 1 remainder
Hence number of odd days in 148 days = 1
Total odd days = 0 + 6+1 = 7
odd days = 0
Hence 28th May 2006 was Sunday.
So the correct answer is option D.
इस प्रश्न को हल करने के लिए हम 28 मई 2006 को छोटे छोटे भागों में विभाजित कर सकते है l
28 मई 2006 को हम (2000 + 5 + 1 जनवरी 2006 + 28 मई 2006) लिख सकते है l
2000 = 1600+400 = 0 विषम दिन + 0 विषम दिन = 0 विषम दिन
अब हम 2000 से 2005 तक विषम दिनों की संख्या ज्ञात करेंगे।
5 वर्ष में विषम दिन = 4 साधारण वर्ष + 1 लीप वर्ष (2000 से 2005 के बीच 2004 लीप वर्ष है)
साधारण वर्ष में विषम दिन = 1
लीप वर्ष में विषम दिन = 2
5 वर्ष में विषम दिन = 4*1 + 1*2 = 4+2 = 6 विषम दिन
1 जनवरी 2006 से 28 मई 2006 तक कुल दिनों की संख्या
जनवरी+फरवरी +मार्च+अप्रैल+28 (मई के दिन)
31 +28+31+30+28 = 148 दिन
148 दिनों में विषम दिन = 148/7 = 21 + 1 शेषफल
अतः 148 दिनों में विषम दिनों की संख्या = 1
कुल विषम दिन = 0 + 6+1 = 7
विषम दिन = 0
अतः 28 मई 2006 को रविवार था l
इसलिए सही उत्तर विकल्प D है l
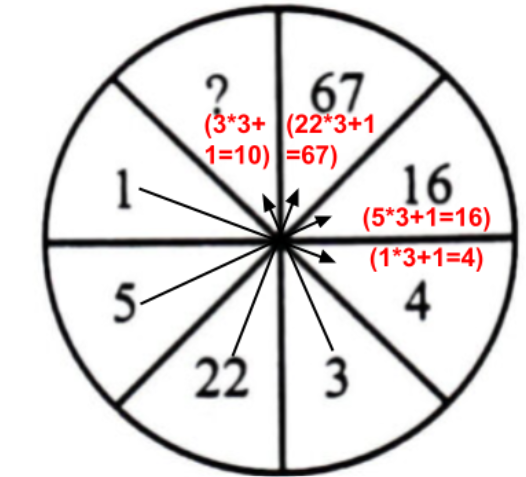
Find out the missing number:
लुप्त संख्या ज्ञात कीजिये:
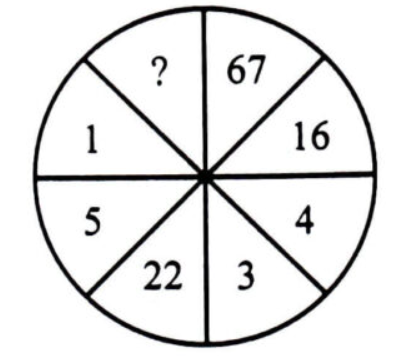
The missing number is 10.
So the correct answer is option C.
You can see its solution in the picture below.
लुप्त संख्या 10 है।
इसलिए सही उत्तर विकल्प C है।
इसका समाधान आप नीचे दिए गए चित्र में देख सकते है।
From the given figures, select the figure which is hidden/embedded in the question figure.
दी गयी आकृतियों में से उस आकृति को चुनिए जो प्रश्न आकृति में निहित है l

The figure given in Option D is embedded in the question figure.
So the correct answer is option D.
विकल्प D में दी गयी आकृति प्रश्न आकृति में निहित है।
इसलिए सही उत्तर विकल्प D है।
2, 3, 10, 38, 172 Find out the wrong number in the series.
2, 3, 10, 38, 172 श्रृंखला में गलत संख्या ज्ञात कीजिए।
2, 3, 10, 38, 172
2 × 1 + 12 = 3,
3 × 2 + 22 =10,
10 × 3 + 32 = 39,
39 × 4 + 42 = 172
Hence 38 is the wrong number and in place of 38, it should be 39. So the correct answer is option A.
2, 3, 10, 38, 172
2 × 1 + 12 = 3,
3 × 2 + 22 =10,
10 × 3 + 32 = 39,
39 × 4 + 42 = 172
अत: 38 गलत संख्या है और 38 के स्थान पर 39 होनी चाहिए। इसलिए सही उत्तर विकल्प A है।
In the given question, select the related letters from the given alternatives.
C-3, E-5, G-7, I-9, ?,?
दिए गए प्रश्न में, दिए गए विकल्पों में से संबंधित अक्षरों को चुनिए।
C-3, E-5, G-7, I-9, ?,?
In the series, each letter is given its alphabetical series number and one letter is skipped in between.
C-3,
E-5,
G-7,
I-9,
K-11
M-13
So the missing letters and number are K-11, M-13.
Hence the correct answer is option A.
श्रृंखला में, प्रत्येक अक्षर को उसकी वर्णमाला श्रृंखला संख्या दी गई है और बीच में एक अक्षर को छोड़ दिया गया है।
C-3,
E-5,
G-7,
I-9,
K-11
M-13
लुप्त अक्षर और संख्या K-11, M-13 हैं।
अतः सही उत्तर विकल्प A है।
In each of the following questions, select the related letter from the given alternatives.
voyage: Sea sickness:: Height:?
दिए गए विकल्पों में से संबंधित शब्द/अक्षर/संख्या चुनिए ।
जहाजी यात्रा: जहाजी मतली: ऊंचाई:?
Just as a voyage causes Sea sickness, similarly, climbing at a height causes Giddiness.
So the correct answer is option C.
जिस प्रकार जहाजी यात्रा करने से जहाजी मतली होती है उसी प्रकार ऊंचाई पर चढ़ने से चक्कर (सिर में) आने लगता है l
इसलिए सही उत्तर विकल्प C है l
Option C will replace the question mark (?).
So the correct answer is option C.
विकल्प C प्रश्नवाचक चिन्ह (?) का स्थान लेगा।
इसलिए सही उत्तर विकल्प C है।